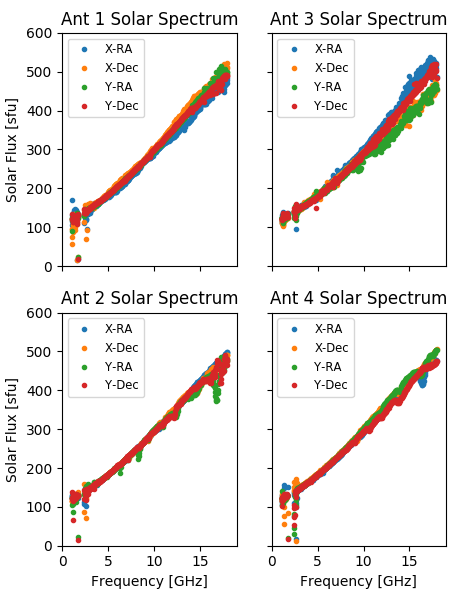
2.1-m Antennas

Az-El Mount 2.1 m Dishes

Testing RF Spin Feed

On 2023 Mar 12 we are testing a new feed using antenna 3. This feed is manufactured by RF Spin, and is a Quad-Ridge Flared-Horn (QRFH) feed. This dual-polarization feed is rated for 1-18 GHz, but the beam gets quite narrow at higher frequencies, so we can expect under-illumination at the higher frequencies.

Pointing/Focus Procedure

Today is the first test of the feed on an antenna, so our procedure will be to first mount the feed (orientation is 90-degrees uncertain, but that can be determined by cross-correlation with other dishes), then do a SOLPNTCAL to check pointing, update the pointing (possibly for several iterations), then attempt to adjust focus. At the end of that procedure, we should have a good idea of the pointing performance vs. frequency. I am hoping to find that the pointing (primary beam) is very stable and uniform over frequency.

The feed has been mounted and both a GAINCAL and SOLPNTCAL have been done. It seems we also need a SKYCAL although I thought the code would allow that to be missing. I'll try to fix this later, but for now I also ran a SKYCAL. The calibration now completes.

Explanation for apparently strange measurements. (a) When near the meridian, and RA/Dec sweep is aligned 45 degrees from the E-plane of the feeds so both sweeps and both feeds give similar beam sizes. (b) When well past the meridian, the RA/Dec axes are tilted relative to the AzEl-mounted feeds, so the RA sweep gives a narrow X beam and a wide Y beam while the Dec sweep gives the opposite behavior.

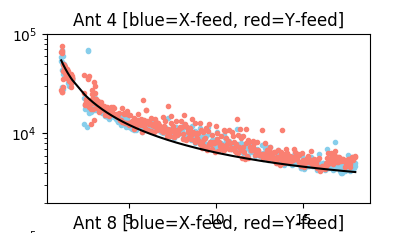
1. The result of the first observation, filename IDB20230312165723, is that the beamwidth is very broad, around twice the nominal primary beamwidth. This might be due to being way out of focus, or it may be the under-illumination. We have now moved the feed about 1" (2.5 cm) farther from the dish.

• 

  Test 1 X_polarization beam size for ants 1-4.

• 

  Test 1 Y_polarization beam size for ants 1-4.

• 

  Test 1 X_polarization pointing offset for ant 3.

• 

  Test 1 Y_polarization pointing offset for ant 3.

• 

  Test 1 Cal factor plot for ants 1-4.

2. The result of the second observation, filename IDB20230312200219, is that the beamwidth is much closer to nominal (!), but strangely the pointing behavior is different for the X feed than for the Y feed. But I'll try moving the feed another 0.5" (1.25 cm) farther and see what happens.

• 

  Test 2 X_polarization beam size for ants 1-4.

• 

  Test 2 Y_polarization beam size for ants 1-4.

• 

  Test 2 X_polarization pointing offset for ant 3.

• 

  Test 2 Y_polarization pointing offset for ant 3.

• 

  Test 2 Cal factor plot for ants 1-4.

3. The result of the third observation, filename IDB20230312205319, is that the beamwidth in one axis is broad again, so things seem to have gotten worse relative to the second observation. I am going to try to split the difference between the first two, i.e. move the feed 0.75" toward the dish relative to its current position.

• 

  Test 3 X_polarization beam size for ants 1-4.

• 

  Test 3 Y_polarization beam size for ants 1-4.

• 

  Test 3 X_polarization pointing offset for ant 3.

• 

  Test 3 Y_polarization pointing offset for ant 3.

• 

  Test 3 Cal factor plot for ants 1-4.

4. The result of the fourth observation, filename IDB20230312213919, is worse in beamwidth on some axes, so I will go back away from the dish again. I got a bit lost in positioning the feed. I think it will be a bit farther out from the dish than the 1" position, so intermediate between 1" and 1.5".

• 

  Test 4 X_polarization beam size for ants 1-4.

• 

  Test 4 Y_polarization beam size for ants 1-4.

• 

  Test 4 X_polarization pointing offset for ant 3.

• 

  Test 4 Y_polarization pointing offset for ant 3.

• 

  Test 4 Cal factor plot for ants 1-4.

5. The result of the fifth observation, filename IDB20230312222119, is still a large beamwidth on some axes. It is not clear to me what might be the cause of this. The gaussian fits look good, but the widths are clearly varying a lot on X feed RA and Y feed Dec. The sizes for the other axes are much narrower and very steady.

• 

  Test 5 X_polarization beam size for ants 1-4.

• 

  Test 5 Y_polarization beam size for ants 1-4.

• 

  Test 5 X_polarization pointing offset for ant 3.

• 

  Test 5 Y_polarization pointing offset for ant 3.

• 

  Test 5 Cal factor plot for ants 1-4.

2023 Mar 13 Focus Tests

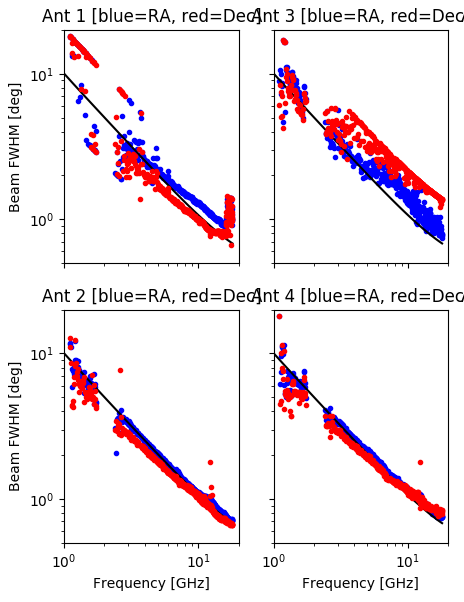
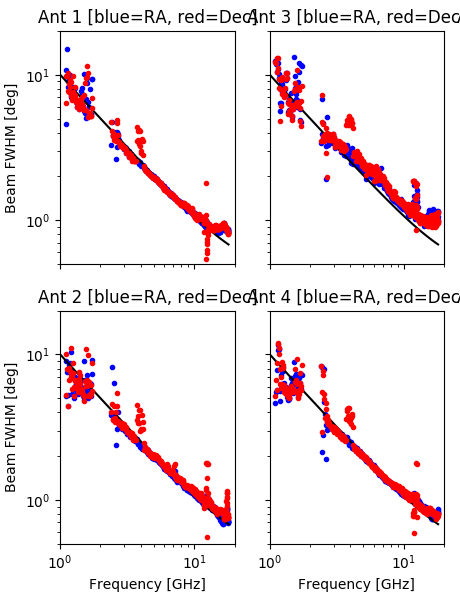
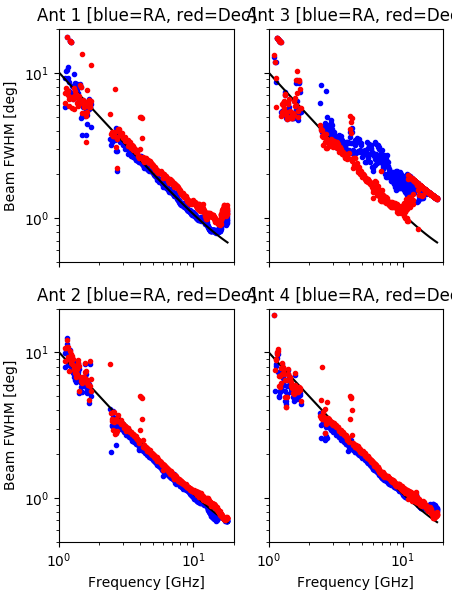
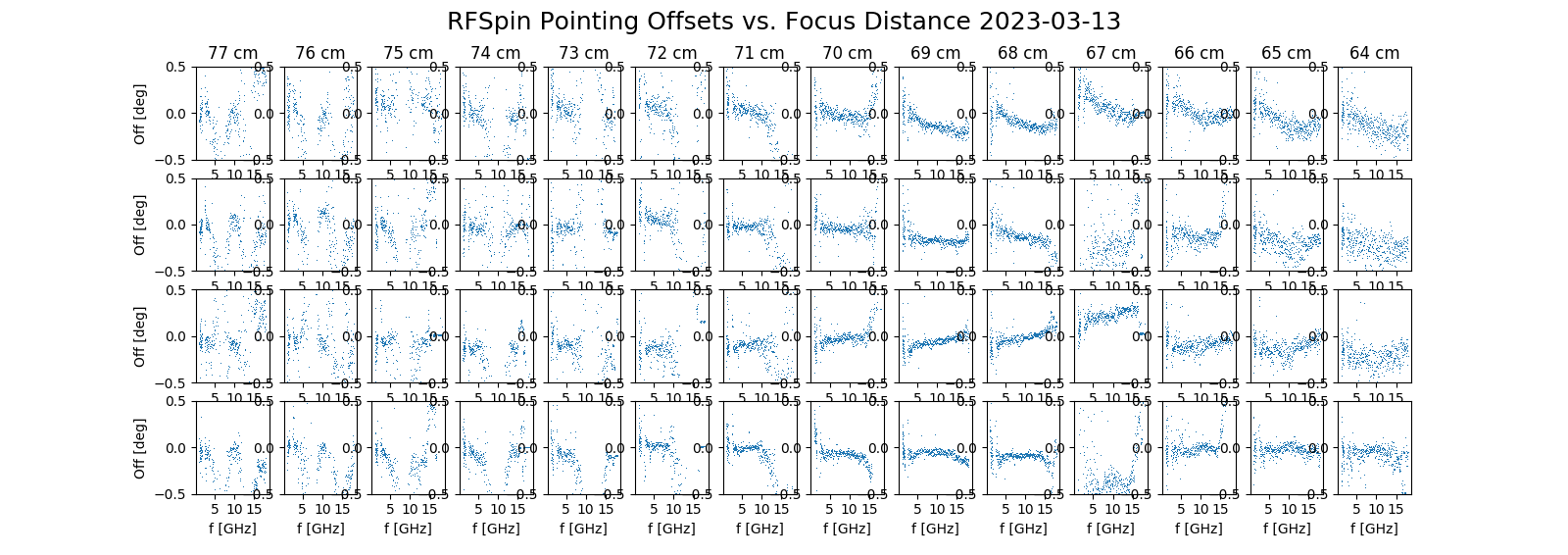
We have figured out that the weirdness of the previous tests was that we were doing an RA-Dec cross pattern but the feeds are moving on an AzEl mount, so their orientation was changing for each test relative to the search pattern. This is coupled with a VERY different beam size in the parallel and cross orientations, so the axis that was affected most kept moving. I have arranged to do the cross pattern in AzEl, and now we are consistently getting the same beam size for both feeds, and it should not change with time. So I am hopeful that a new focus search will give more consistent and comparable results.

We are doing a new series of tests where the focus distance will start at the position farthest from the dish and move in (toward the dish, so farther out on the studs) 1 cm at a time. Rather than doing the analysis between tests, which is quite time consuming, we will just do all of the tests at once, one after another, and I'll do the analysis afterwards.

Test 1: 20:24:57 77 cm from dish

Test 2: 20:40:59 76 cm from dish

Test 3: 20:59:13 75 cm from dish

Test 4: 21:10:11 74 cm from dish

Test 5: 21:21:16 73 cm from dish

Test 6: 21:33:01 72 cm from dish

Test 7: 21:44:31 71 cm from dish

Test 8: 21:56:25 70 cm from dish

Test 9: 22:07:11 69 cm from dish

Test 10: 22:18:21 68 cm from dish

Test 11: 22:30:39 67 cm from dish

Test 12: 22:43:11 66 cm from dish

Test 13: 23:04:55 65 cm from dish

Test 14: 23:18:16 64 cm from dish

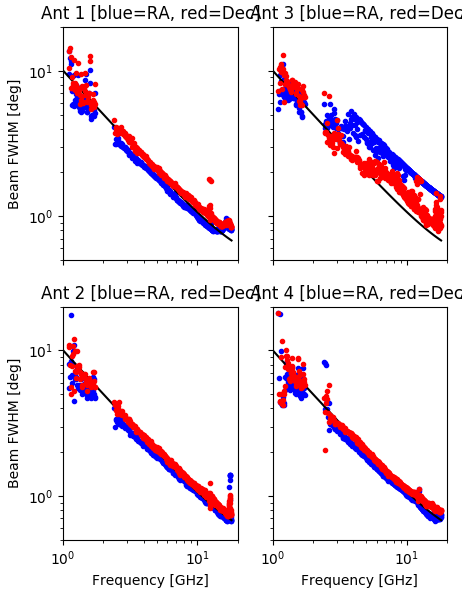
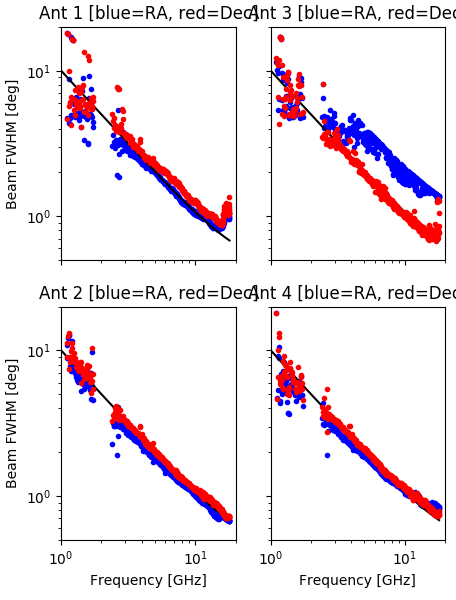
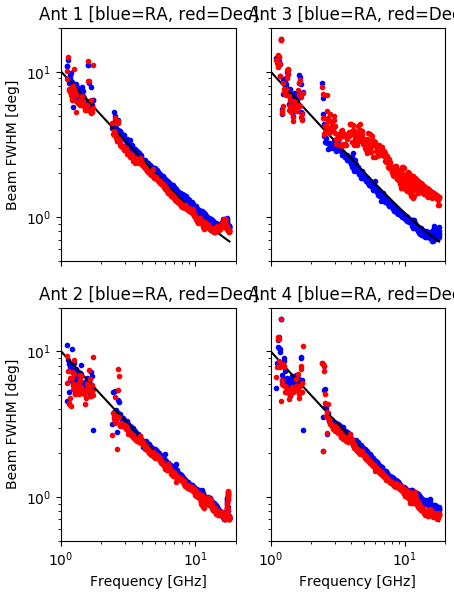
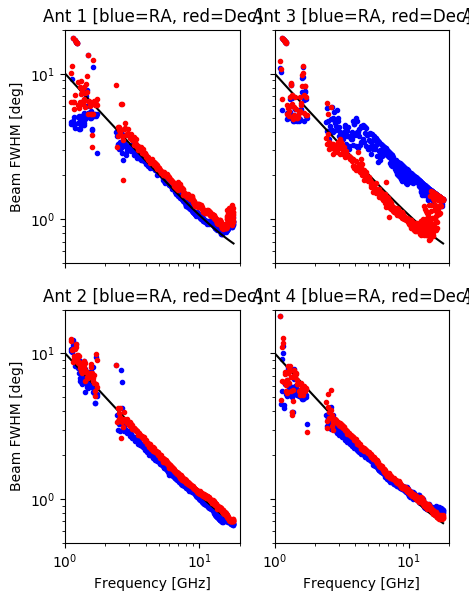
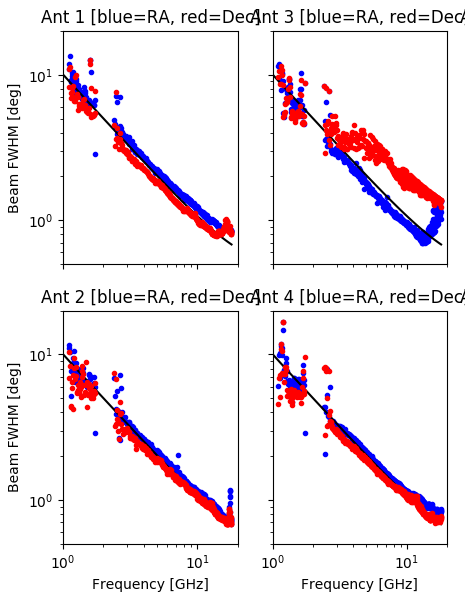
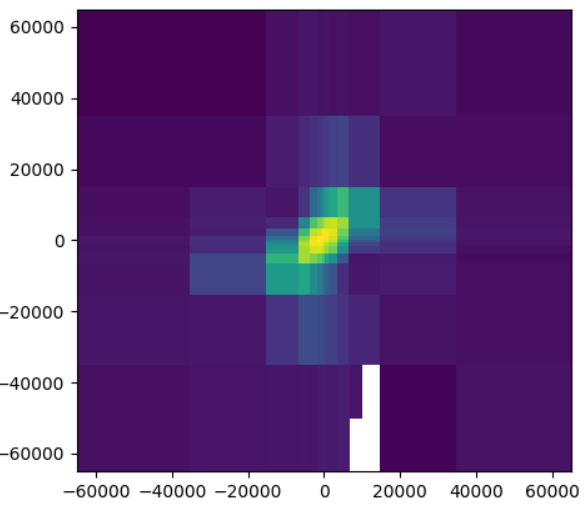
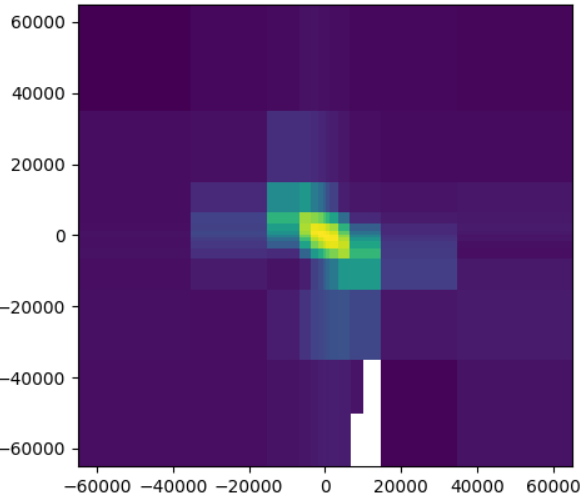
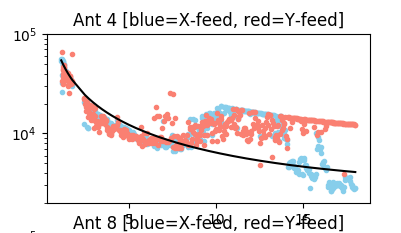
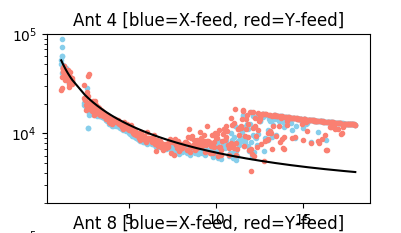
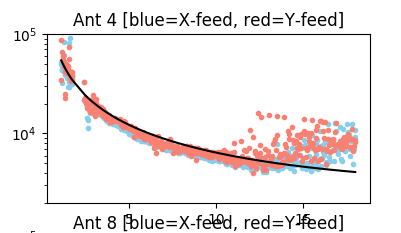
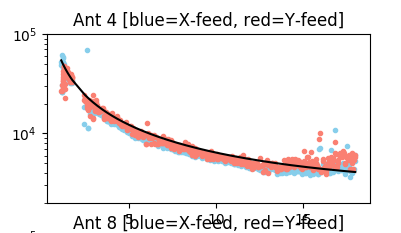
Results: The results are shown in the two figures above and are quite regular and understandable. The best focus is clearly around 69 cm from the dish, with the beamsize being larger than nominal in both axes. However, the earlier tests clearly show that the E-plane feed beamwidth is larger on the dish (illuminates more of the dish), and hence smaller on the sky, so the beamsize in that direction is more or less nominal. The B-plane feed beamwidth is smaller on the dish (illuminates only the central part of the dish), and hence nearly twice as large as the nominal size on the sky.

Phase Calibration

Once the best pointing and focus have been found, we will observe a calibrator and check the delays. I tried this on 2023 Mar 12 when the focus was not correctly set, in the file IDB20230312224929. This resulted in an "okay" delay without modification, which is interesting. However, when I plotted the Ant 14 - Ant 3 phase it was not flat with frequency, but showed the characteristic U shape indicating a change in feed phase center vs. frequency. The U was much less pronounced than that of the Tecom feeds relative to the 27-m feed horns, so presumably the feed phase center has less of a meander than the Tecom feeds. This exercise needs to be repeated, but is postponed until tomorrow (2023 Mar 15) due to rain today.

2023 Jul 22 Tests of Feed with Radome

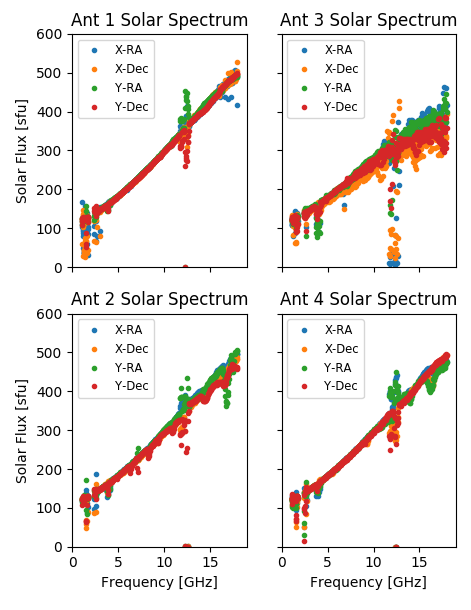
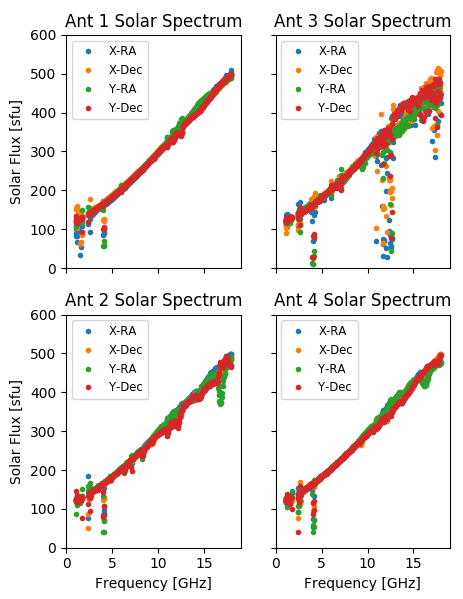
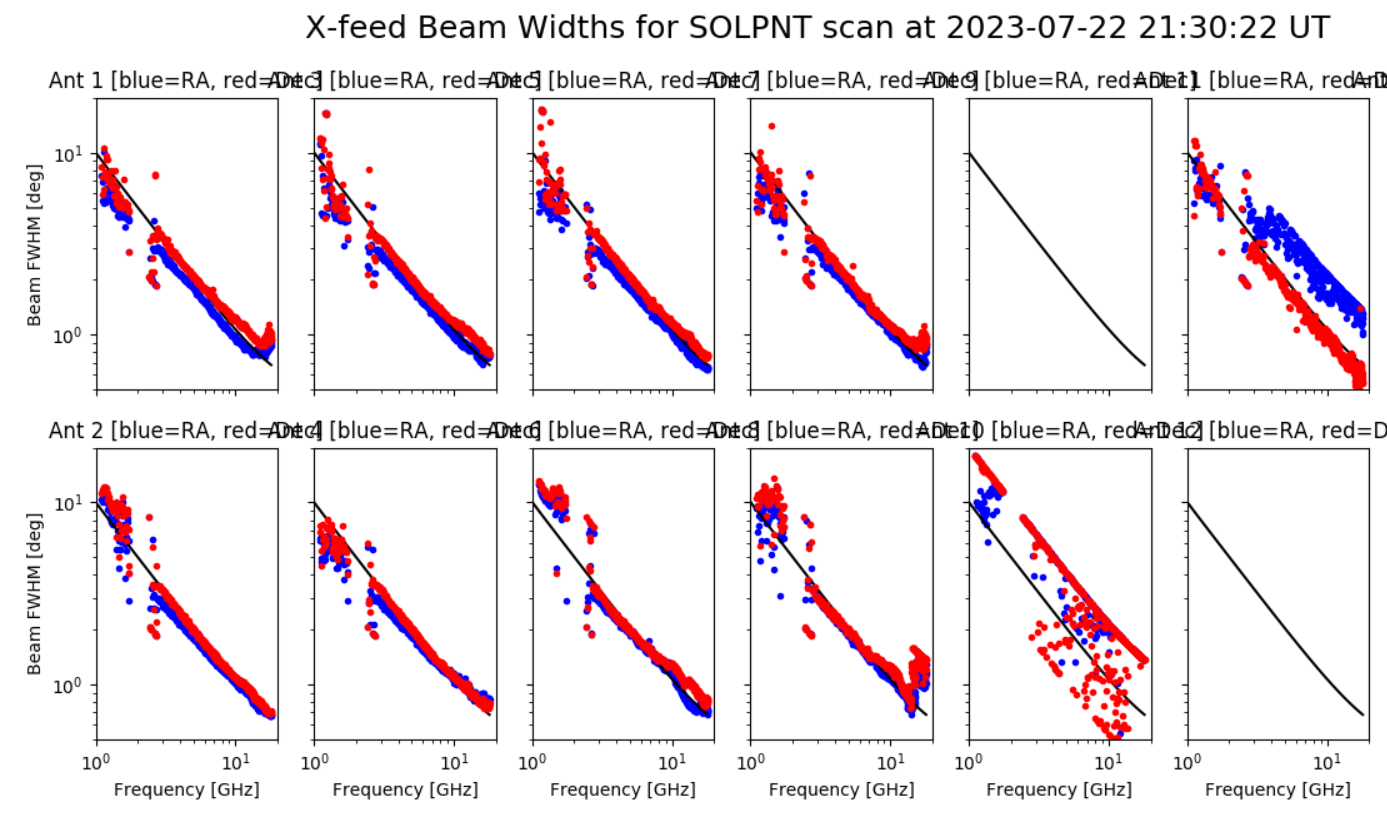
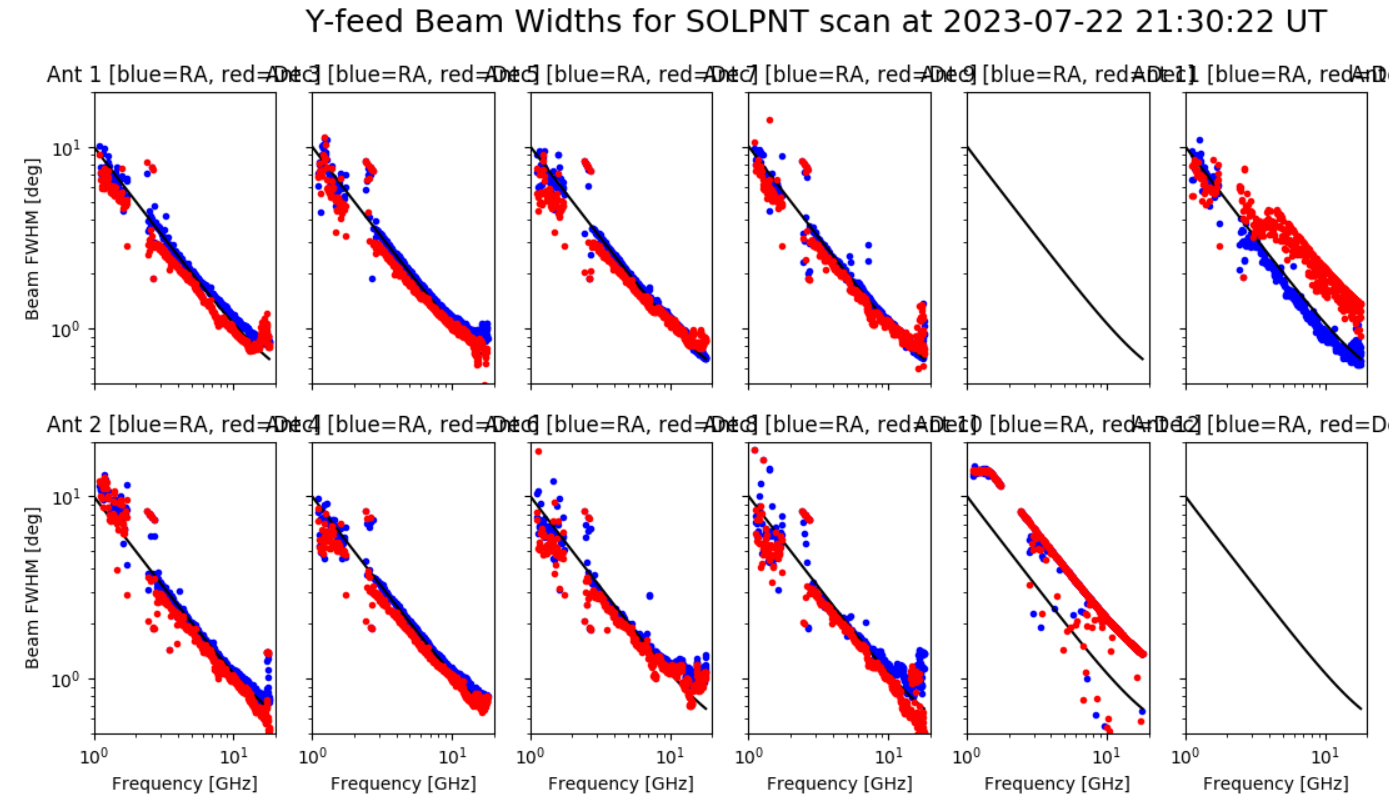
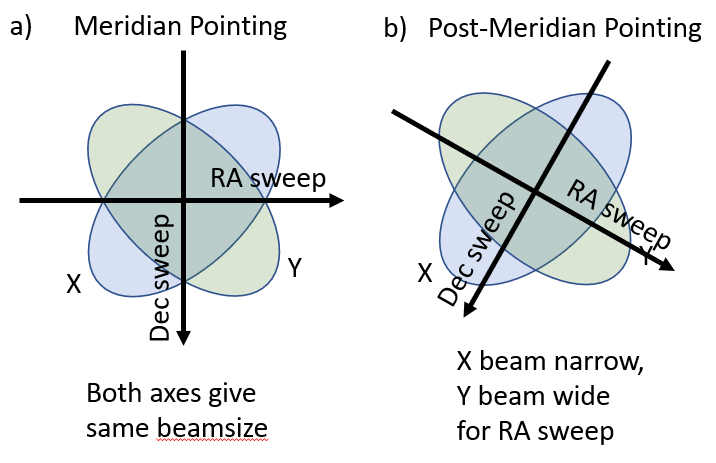
On 21 July, Owen got our new radome-equipped feed mounted on Ant 11, so I spent 21 and 22 July working on pointing and delays. The feed seems to be performing nominally, with the expected oval-shaped beam that is twice as wide in the B plane (because the feed pattern is twice as narrow, which under-illuminates the dish). Since Ant 11 is an equatorial mount, it is not necessary to use the Az-El sweep pattern to measure the beam size. Below are the results after adjusting the pointing. Note that this measurement indicates that the feed is rotated 45 degrees from what it should be, showing the maximally different beam sizes for orthogonal directions of a + pattern instead of the roughly equal responses expected of an X pattern.

• 
• 

Pointing in the Era of RF Spin Feeds

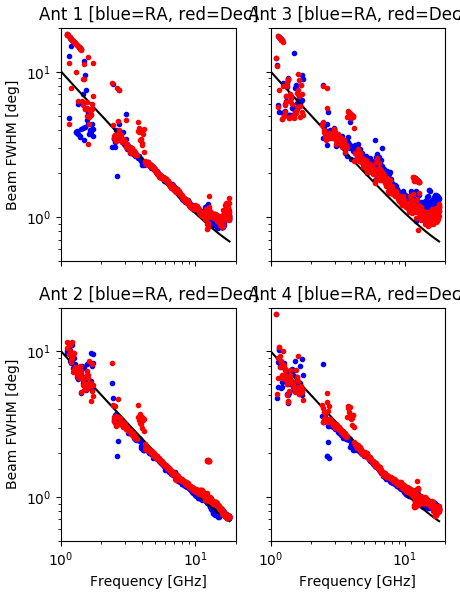
We have purchased 13 RF Spin feeds equipped with radome. These feeds are to replace the many burnt feeds, and we will fit out the entire array with them. However, their elongated feed pattern is causing problems with determining optimum pointing using our current scheme, and so they require a new method of pointing calibration. The problem stems from the fact that the feed pattern center (x direction, say) shifts as the other (y coordinate) changes, so determining a center from a simple RA-Dec cross pattern doesn't work. As a check, I performed a grid of off-point measurements that demonstrates the highly elliptical feed pattern as shown below for the feed on Ant 11.

• 
• 

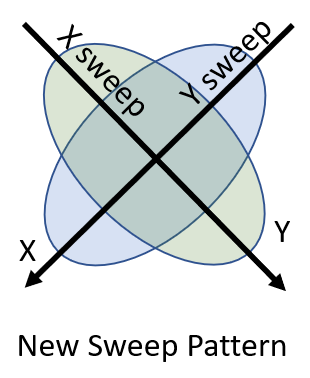
A better way to do the search is to rotate the cross pattern to align with the feed axes (which are in an X shape relative to their coordinate axes) as shown in the figure at right.

New Pointing Search Pattern. Sweeping in this way means that the center of the pattern in one dimension remains in the same place as the offset in the other dimension changes. This will also provide a stable measurement of the beam widths in the two orthogonal directions for each polarization.

The problem is that this alignment needs to be done with offsets in RA-Dec for the equatorial dishes and in Az-El for the AzEl dishes. Also, the analysis of the data becomes a bit more complicated. But since this is obviously the right thing to do, I will proceed with the idea. Taking the data will be easy enough using the already established means of creating offsets in a trajectory file, sending it to the Az-El dishes via a file with a .azel extension, and to the equatorial dishes via a file with a .radec extension. However, the Az coordinates should be increased by Az/cos(El), and the RA coordinates should be increased by RA/cos(Dec), so these would have to be calculated for the date/time in question. That means writing a routine to create the files on the fly. The analysis is not that different from what I do now, but with a few important changes such as calculating the vector offset length and keeping track of the fact that RA and Az offsets have opposite signs!

For specificity, note that the X pattern is done starting with offsets in (-Az, +Dec), sweeping to (+Az, -Dec), then starting with (+Az, +Dec), sweeping to (-Az, -Dec). For the older antennas, the -Az corresponds to +RA and vice versa, i.e. (+RA, +Dec) to (-RA, -Dec), etc., so that the patterns are identical at the meridian. The magnitude of the offsets is 10, 5, 2, 1, 0.5, 0.2, 0.1, 0, -0.1, -0.2, -0.5, -1, -2, -5 degrees for each sweep.

More Focus Tests (2023-Dec-05)

antenna   focus posn    start time
 ANT 4     11.5 cm       18:15 UT
 ANT 4      8.5 cm       20:26 UT
 ANT 4      9.5 cm       20:38 UT
 ANT 4     10.5 cm       20:50 UT
 ANT 4     12.5 cm       21:03 UT
 ANT 4     13.5 cm       21:18 UT
 ANT 4     14.5 cm       21:34 UT

• 

  Focus position 8.5cm

• 

  Focus position 9.5cm

• 

  Focus position 10.5cm

• 

  Focus position 11.5cm

• 

  Focus position 12.5cm

• 

  Focus position 13.5cm

• 

  Focus position 14.5cm

Equatorial Mount 2.1 m Dishes

EOVSA comprises 8 newer azimuth-elevation-mounted 2.1 m dishes (plus currently a 9th one, the South Pole dish), and 5 older equatorial-mounted 2.1 m dishes. This document describes some of the important differences for these older dishes.

Parallactic angle

The equatorial mounts were outfitted with the same reflector as used for the newer dishes, so that they function in the same way, except that their feeds are fixed in orientation on the sky while the feeds on the newer azel dishes rotate due to the parallactic angle. This angle is computed by the schedule (in stateframe.py), for the current pointing coordinates of each antenna, and inserted into the stateframe as Sche_Data_Chi (SQL naming convention), or sf[‘Schedule’][‘Data’][‘Chi’] (python naming convention), defined as the angle of the azel dish feed relative to an equatorial mount. It should be noted that it is calculated for all antennas, independent of whether the dish is an azel or equatorial mount. For a given azimuth and elevation, the paralactic angle is computed from

$\chi =\arctan 2(-\cos \lambda \sin a,\sin \lambda \cos e-\cos \lambda \sin e\cos a)$

where $\lambda$ = latitude (37.233170 degrees for OVRO), $a$ = azimuth, and $e$ = elevation. The arctan2 function resolves the 180-degree ambiguity. Note that any baseline involving two dissimilar dishes, the phase will rotate according to the parallactic angle, and will need to be corrected by the DPP prior to writing to the Miriad database. The default phase will be that of the azel dishes—that is, baselines with one azel and one equatorial dish will be phase-corrected to correspond to the phase as measured by two azel dishes. [This statement will need to be tested, and possibly amended if it is not correct.]

Pointing of the equatorial-mount dishes—step size

The equatorially-mounted dishes have a step-motor drive system, consisting of a motor of s = 200 steps/revolution, followed by a harmonic drive (a complication is that we have two DIFFERENT harmonic ratios in use, three dishes with h = 100:1 and two with h = 160:1). These motors drive a 20-tooth sprocket gear and meshes with a chain having the equivalent of 225 “teeth” in one revolution, for a further reduction r = 225:20. In addition, we are running the motors with a 16:1 microstepping ratio ($\mu$), which means that 16 microsteps are needed for one motor step. It is these microsteps that are counted by the system. The resulting of microsteps/degree, then, is

$n=\mu shr/360$

This makes a nice round number, n = 10000 steps/degree for h = 100:1, and n = 16000 steps/degree for h = 160:1. Currently, Ants 9, 11 and 13 have 10000 steps/degree, while Ants 10, and ultimately 12 will have 16000 steps/degree. These values are given in the crio.ini file. Obviously, these are nominal values, and the true step size could be slightly different. The step size needs to be part of the pointing parameter solution.

Pointing of the equatorial-mount dishes—restricted sky coverage

The equatorial-mount dishes have a restricted sky coverage relative to the azel dishes, given in terms of hour angle limits and declication limits. The precise limits (prior to any pointing corrections) can be determined by adjusting the “hard limits” (limit switches) to trigger just before the antenna hits the stops, and reading the angles at those stopped points. In order to achieve the greatest sky coverage, the hard limits should be set as close as possible to the stops, but with due regard for possible collisions of cables and other obstructions by the mount. In particular, the thick conduit on the north side of the mounts can interfere with the counter-weights when close to the stops, so the limit switches must be set somewhat away from the stops to allow the counter-weights to clear. This has been done with some care on antennas 9 and 10, with the following results (by way of example). The “soft limits” are then selected to stop the motion programmatically just before the limit switch would trigger. It is very important that the motors never reach the hard stops, since that causes the motor to stall while still counting, and hence the step count is compromised. The pointing is only good if the step count is known.

Axis	Ant 9 Hard Limit	Ant 9 Soft Limit	Ant 10 Hard Limit	Ant 10 Soft Limit
HA Low	-59.81	-59.5	-58.7	-58.0
HA High	+58.33	+58.0	+59.3	+59.0
Dec Low	-24.28	-24.0	-24.27	-24.0
Dec Hi	+45.43	+45.0	+46.25	+46.0

One concern is that, once the pointing coefficients (and step size) are determined, the angular positions shift somewhat. It would be better to have these hard- and soft-limits not change just because of pointing coefficient adjustments. This will require some thought. One consequence of this restricted sky coverage is that there will be times (especially in the summer) when some of the dishes cannot reach the Sun or calibrator. With the current set up, when a position is requested that cannot be reached by a dish, it will go as close to the position as possible and then just wait there. The position error can be used to determine which dishes are not tracking. For calibration, all calibrator sources will need to be chosen to respect the equatorial dishes, however, since the 27-m antennas also have this same sky coverage limitation. Therefore, the schedule, which chooses the “best” calibrator automatically, must be set to use the above sky coverage limitation. Another, rather serious consequence of the restricted sky coverage is that the SOLPNTCAL procedure, which currently runs twice per day, works by off-pointing the dishes by +/- 5 degrees from the Sun in both RA and Dec. Since the south limit of the dishes is only -24 degrees, the dishes will not be able to reach -5 degrees from Sun center whenever the Sun is below declination -19 degrees. This is a date range of roughly Nov 18-Jan 25! During this period, the equatorial-mount dishes will not be able to do a SOLPNTCAL. It could be possible to somehow adjust the procedure to allow some sort of analysis (full HA and half of Dec, for example).

Pointing of the equatorial-mount dishes—star pointing

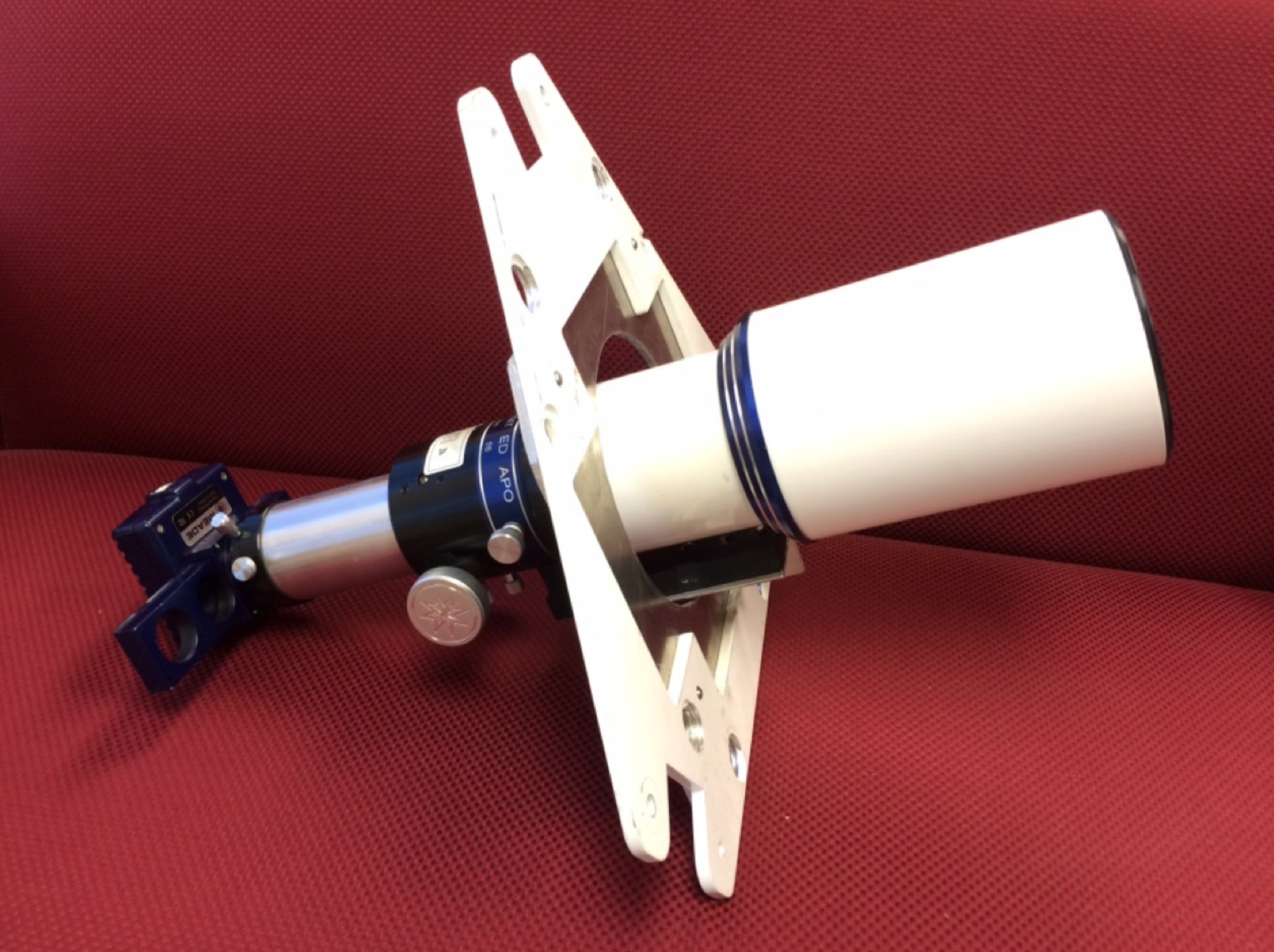
I made an attempt to observe stars with Ant 9, but was not happy with the constant vibrations, which cause the stars to be linear rather than round. I discussed it with Kjell, and he had a new mount for the telescope made (Figure 1), which will go in place of the feed package. With luck, this should allow for much less vibrational motion and hence result in much better star images. I plan to do a first test on Ant 9 tonight.

Figure 1: The new mount for the optical telescope, to be put in place of the radio front-end receiver. This should greatly reduce vibrations that lead to non-circular stars.

In addition, I updated the startracktable() routine in readbsc.py to account for the reduced sky coverage of the equatorial-mount dishes, since my earlier attempt did not do this, and the antenna spent a lot of time at the limits.

Debugging

Ant 12 (the SPASRT antenna) may need its turn count adjusted. To do this, connect to its web page and change parameter 20.16. If its current turn count is 2, set it to 1 and reboot. If it is currently 1, set it to 2 and reboot.