Polarization Mixing Correction (Old): Difference between revisions
No edit summary |
No edit summary |
||
| Line 31: | Line 31: | ||
X_2 \\ | X_2 \\ | ||
Y_2 | Y_2 | ||
\end{bmatrix} | |||
</math></center> | |||
and the Mueller matrix associated with the cross-correlation is found by taking the outer product, i.e. | |||
<center><math> | |||
\begin{bmatrix} | |||
XX' \\ | |||
XY' \\ | |||
YX' \\ | |||
YY' | |||
\end{bmatrix} | |||
= J_1 \otimes J_2 = \begin{bmatrix} | |||
\cos\chi & 0 & \sin\chi & 0 \\ | |||
0 & \cos\chi & 0 & \sin\chi \\ | |||
-\sin\chi & 0 & \cos\chi & 0 \\ | |||
0 & -\sin\chi & 0 & \cos\chi | |||
\end{bmatrix} | |||
\begin{bmatrix} | |||
XX \\ | |||
XY \\ | |||
YX \\ | |||
YY | |||
\end{bmatrix} | \end{bmatrix} | ||
</math></center> | </math></center> | ||
= Status of tests = | = Status of tests = | ||
Revision as of 14:25, 21 October 2016
Explanation of Polarization Mixing
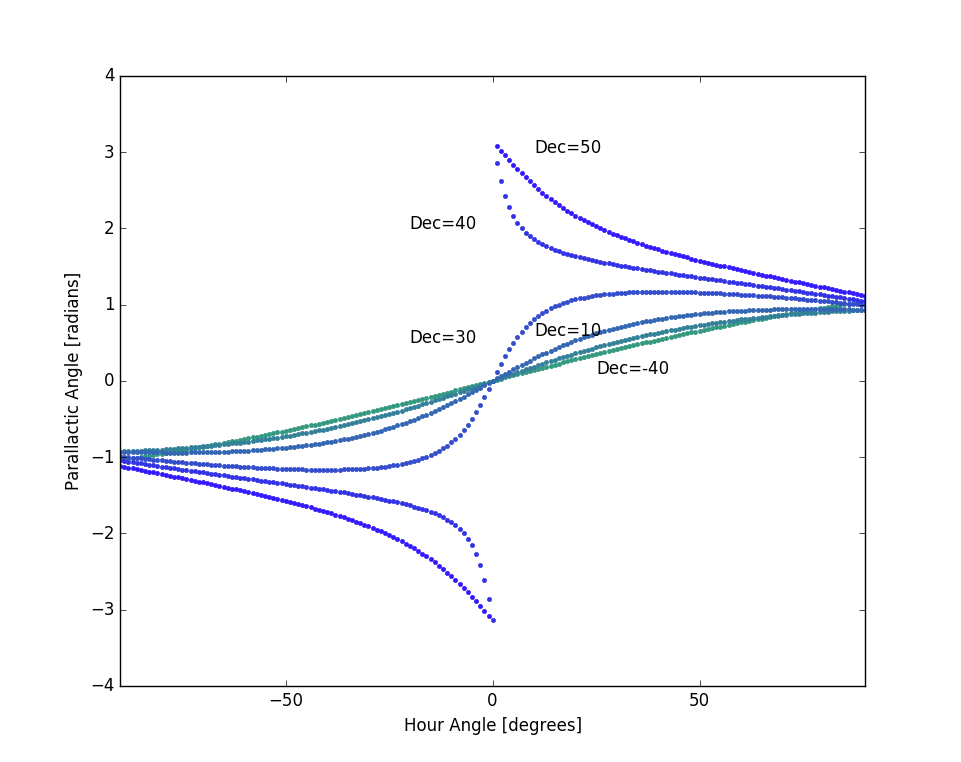
The newer 2.1-m antennas [Ants 1-8 and 12] have AzEl (azimuth-elevation) mounts (also referred to as AltAz; the terms Altitude and Elevation are used synonymously), which means that their crossed linear feeds have a constant angle relative to the horizon (the axis of rotation being at the zenith). The older 2.1-m antennas [Ants 9-11 and 13], and the 27-m antenna [Ant 14], have Equatorial mounts, which means that their crossed linear feeds have a constant angle with respect to the celestial equator, the axis of rotation being at the north celestial pole. Thus, the celestial coordinate system is tilted by the local co-latitude (complement of the latitude). This tilt results in a relative feed rotation between the 27-m antenna and the AzEl mounts, but not between the 27-m and the older equatorial mounts. This angle is called the "parallactic angle," and is given by:
where is the site latitude, is the Azimuth angle [0 north], and is the Elevation angle [0 on horizon]. This function obviously changes with position on the sky, and as we follow a celestial source (e.g. the Sun) across the sky this rotation angle is continuously changing in a surprisingly complex manner as shown in Figure 1. Note that at zero hour angle for declinations less than the local latitude (37.233 degrees at OVRO), but is at higher declinations.
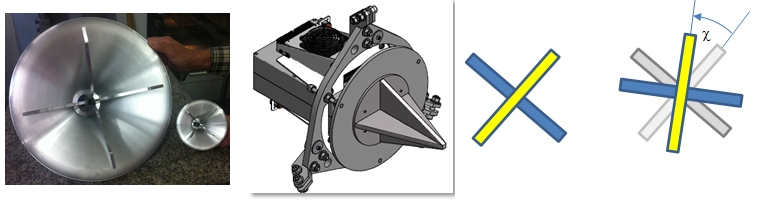
The crossed linear dipole feeds on all antennas are oriented with the X-feed as shown in Figure 2, at 45-degrees from the horizontal, when the antenna is pointed at 0 hour angle. This is the view as seen looking down at the feed from the dish side, although since the feeds are at the prime focus this is the same as the view projected onto the sky. At other positions, the feeds on the AzEl antennas experience a rotation by angle relative to the equatorial antennas.
Because of this rotation, the normal polarization products XX, XY, YX and YY on baselines with dissimilar antennas (one AzEl and the other equatorial) become mixed. The effect of this admixture can be written by the use of Jones matrices. Consider antenna 1 whose feed orientation is rotated by , cross-correlated with antenna 2 with unrotated feed. The corresponding Jones matrices are:
and the Mueller matrix associated with the cross-correlation is found by taking the outer product, i.e.